To do this part of the lesson, download this Geometer's Sketchpad file and open it.

Recall from the previous section that you found the SIN of an angle to be the ratio of the OPPOSITE side to the HYPOTENUSE. As you may have thought there are ratios that can be formed using the other sides of the triangle. In this section, we will investigate the other major types of trig ratios.

In the Sketchpad file we have three circles all with radii of 1. Each is called the UNIT CIRCLE. We will use it to investigate the other trig ratios.

STEP 1: Consider this diagram.

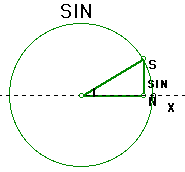
Look at triangle NIS. The HYPOTENUSE is the same as the radius of the circle. Therefore, it has a measure of 1. Recall the SIN ratio we discovered in the last section for angle SIX. It is,

SIN (< XIS) = OPPOSITE (SN) / HYPOTENUSE(IS)

Since the HYPOTENUSE = 1, the SIN (angle XIS) = the length of the OPPOSITE side. That's what makes the UNIT CIRCLE special!

<>STEP 2:MEASURE <SIX, MEASURE the SIN of <SIX,  <>Now, measure the length of SN?
What do you notice about the measures? Explain why this is so.

STEP 3:Double-click the ANIMATE button under the SIN circle.

Form a conjecture about the relationship between the side length (SN) and the SIN of the angle.

For the next steps use the circle under the COS label.

<>STEP 4:MEASURE <XOC, MEASURE the cosine (COS) of <XOC,  <>Now, measure the length of OS?
What do you notice about the measures? 

STEP 5:Double-click the ANIMATE button under the COS circle.

Form a conjecture about the relationship between the side length (OS) and the COS of the angle.

STEP 6: Write down the ratio for the COS <XOC 

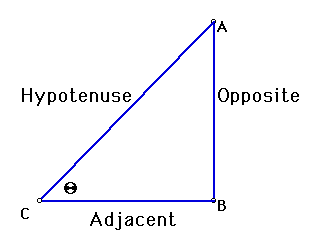
STEP 7: Use the figure below to write down the ratio for the COS <BCA 

For the next steps use the circle under the TAN label.

<>STEP 8:MEASURE <TAX, MEASURE the Tangent (TAN) of <TAX,  <>Now, measure the length of TA, TN, and AN?
Do you notice anything about the measures of the sides and the TAN <TAX? 

CALCULATE the ratio of SIDE (TN) to SIDE (AN) 

Now, do you notice anything about the measures of the sides and the TAN <TAX? 

STEP 9:Double-click the ANIMATE button under the TAN circle.

Form a conjecture about the relationship between the side lengths (TN) and side (AN) and the TAN of the angle.

STEP 10: Write down the ratio for the TAN <TAX 

STEP 11: Use the figure below to write the ratio for the TAN <BCA