The Problem
Problem Description
You currently work for your hometown's fire department. They have
placed you in charge of buying a new ladder for the department. You
need to find out, how tall a ladder to purchase so that you'll be able
to reach the top of any building in town. The tallest building is ten
stories high. How tall a ladder do you have to buy?

In this module students will,
• investigate
trigonometric ratios
• examine
the graphs of simple trig functions
• investigate
the connections between various trig identities
To be able to find the height of the ladder that you need to
buy, it is necessary to use trig. Our problem can be described in the
following picture.
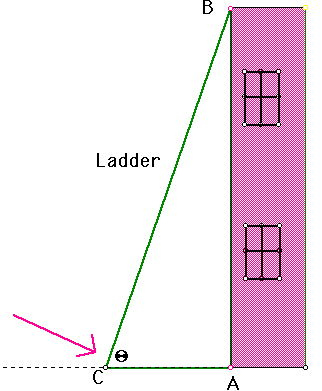
The above diagram shows the building and a ladder leaning
against it. The height of the building is 10 stories (remember that a
story is equal to 10 feet). Therefore, the building is 100 ft
tall. Notice how the ground, the building and the ladder form three
sides of a triangle.
Questions to think about:
1. Why don't we just buy a 100 foot ladder?
2. Think about the Pythagorean Theorem and how it could be used for
this problem.
To help us solve this problem, we are going to use a
Geometer's Sketchpad file. If needed a short tutorial is provided if
the student is unfamiliar with Geometer's Sketchpad.
GSP - Tutorial.
Otherwise, Continue the Lesson
with Worksheet 1.



