Before we use Sketchpad to help us solve this problem, let's take a trip to the hardware store.

At the store they sell a couple different types of ladders. They don't sell them 100 ft long, so we'll have to custom order it. You find out that it costs 2$/ft for the ladder. Also, you read a label on a ladder that says

The ladder can only be safely used if the angle of the ladder to the ground is no more than 75 degrees!. This can be demonstrated by the picture below.

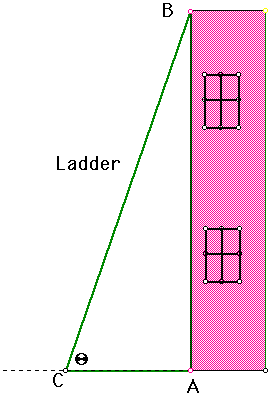
Notice how the ladder, building and ground make up three sides of a triangle.

To do this part of the lesson, download this Geometer's Sketchpad file.

Open the Sketchpad file and follow the following steps.

The first thing we need to is to make the angle the ladder forms with the ground to 75 degrees.

STEP 1: MEASURE < BCA (SELECT the points B, C and A in order and go to the MEASURE menu and select ANGLE.
Write down your measurement.

STEP 2: SELECT and MOVE point C so that the angle you just measured is 75 degrees.

We now know the angle measure and one side (the height of the building). Using trigonometry we can now find every angle and side in the triangle.

STEP 3: Double-click the SHOW button on the top of the screen. This will tell you the LENGTH of the LADDER for a 75 degree angle.
Write down the answer.

STEP 4: Select BOTH the measurements (the ladder and the building) and go the menu MEASURE and select CALCULATE....

STEP 5: Under VALUES select the length of the building and then click / (for divide) and finally under values select the length of ladder .
Click OK. Write down your answer

STEP 6: Next, select the angle measurement you found in STEP 1. Under the MEASURE menu, select CALCULATE.....

STEP 7: Under FUNCTIONS select the option SIN( and then under VALUES select < BCA . Click OK. Write down your answer.

STEP 8: What do you notice about the ration you calculated and the SIN of the angle?

It is common to label the sides of a triangle as in the figure below.

NOTE that the side names are dependent on the angle you are considering except for the HYPOTENUSE which is always the side across from the right angle.

STEP 9: Write a ratio using the names of the sides in the picture above to describe the SIN of < BAC.

SIN of < BCA =

-Back to Problem Statement-  -Go to Lesson Worksheet 2-