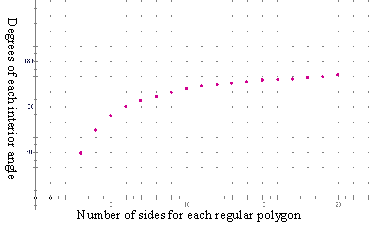Hints:

Time Estimate	Activity	Main Idea
10-15 min (1:20)	Can we graph the relationship?	Graphs can help you visualize relationships.
20 min (1:40)	Discuss the fact that this is a discreet graph. That is, it is not continuous. Why would is be wrong to connect the dots below.

We want to explore different ways to get at the pattern. That is, look at it visually and with variables.

Spend time talking about discreet vs. continuous graphs. Why would it not make sense to connect the points on the graph if we are talking about polygons.

In mathematics a limit is a value that is approached increasingly by a function or a sequence.

This is what the plot on the participant's page should look like.   Notice that the dots do not (and will not) reach 180 degrees. 180 degrees is the LIMIT!

Step-by-step guide

1. Have the participants label the axes. Make the horizontal axis the number of sides and the vertical axis the angle sums.

2. Note that there should only be points. It is misleading to draw lines connecting the points because the values between the points do not have meaning.

Participants may have trouble with labeling graphs since there will be different scales. That is, the vertical axis should have hash marks labeled 180,360, etc. and the vertical will be labeled 3,4,6...

An excel chart with these values is available at https://mste.illinois.edu/m2t2/table2Polygons.xls .